Solid Uniformly Charged Sphere

A Continuous Charge Distribution which is 3 dimensional and filled in with charges:

Properties §

• $\rho$ = Volume charge density (in $C/m^3$)
• $r$ = Radius of sphere (in $m$)

Electric Field §

Assume a uniformly charged sphere (with $\rho$ constant), with total charge $q$ and radius $R$. Let $r$ be the distance from the centre of the sphere. Then the Electric Field is given by:

$$\vec{E}=\{\begin{array}{ll}\frac{k_e\times q}{r^2},& r>R\text{ (outside sphere)}\\ \frac{k_e\times q\times r}{R^3},& r<R\text{ (inside sphere)}\end{array}$$

This means, the electric field linearly increases inside the sphere, and decreases at $\frac{1}{r^2}$ outside the sphere.

Note how if we move outside the sphere, the electric field is the same as the electric field for a point charge!

Derivation With Gauss’ Law §

We know a sphere is symmetrical everywhere, so the electrical field must also be symmetrical. This means the field lines extend radially outward (or inward if the charge is negative).

As such, a suitable Gaussian Surface is a sphere!

$$\Phi =\vec{E}\cdot \vec{A}=\frac{q}{{ϵ}_0}$$

The area vectors of the gaussian surfaces are always parallel to the electric field, due to the charge distribution and the surface being the same shape, so:

$$\Phi =|E||A|$$

$$A_{sphere}=4\pi r^2\text{ (the gaussian sphere!)}$$

Combined, let $r$ = radius of gaussian sphere, and $R$ = radius of charged sphere:

Outside the sphere §

If we are interested in the electric field outside the sphere, we can take a larger gaussian sphere, so the charge enclosed, $q_{enclosed}$ is simply the charge of the sphere, $q$

$$|E|=\frac{q}{{ϵ}_{0}A}=\frac{q}{4{ϵ}_0\pi r^2}=\frac{k_{e}q}{r^2}$$

Inside the sphere §

To find the field inside the sphere, we need a gaussian sphere that’s smaller than the charged sphere. In that case, the charge enclosed can be represented as a ratio of the total charge:

$$q_{enclosed}=q\times \frac{V_{guass}}{V_{sphere}}=q\times \frac{r^3}{R^3}$$

$$|E|=\frac{q_{enclosed}}{{ϵ}_{0}A}=\frac{q{r}^3}{{ϵ}_0\times 4\pi r^2{R}^3}=\frac{q{k}_{e}r}{R^3}$$

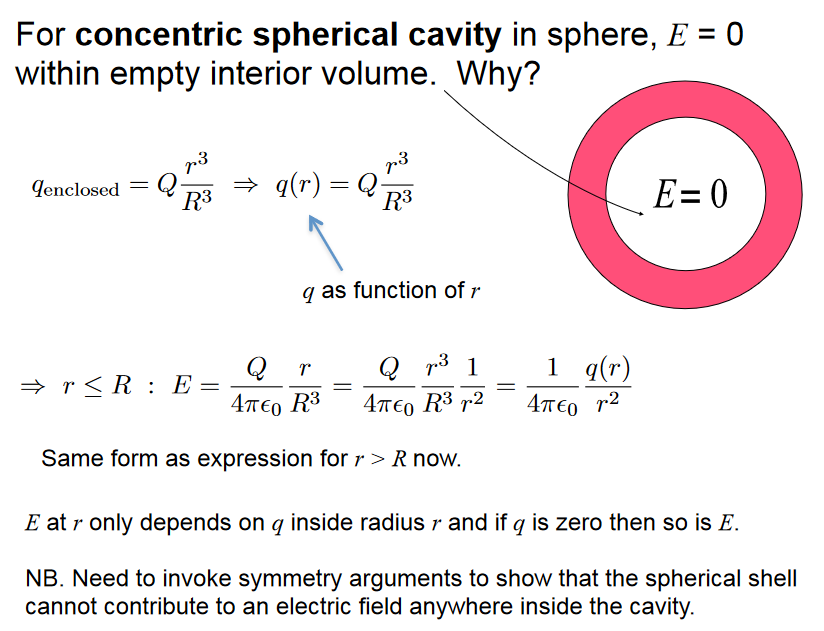
It’s important to note that, due to the gaussian surface being inside the sphere, there’s also a portion of the sphere’s electric charge that affects the surface. However, we can use symmetry arguments to show that it does not matter.#ask

#todo represent both outer and inner radii as a function, like so:

Resources §

• http://hyperphysics.phy-astr.gsu.edu/hbase/electric/elesph.html