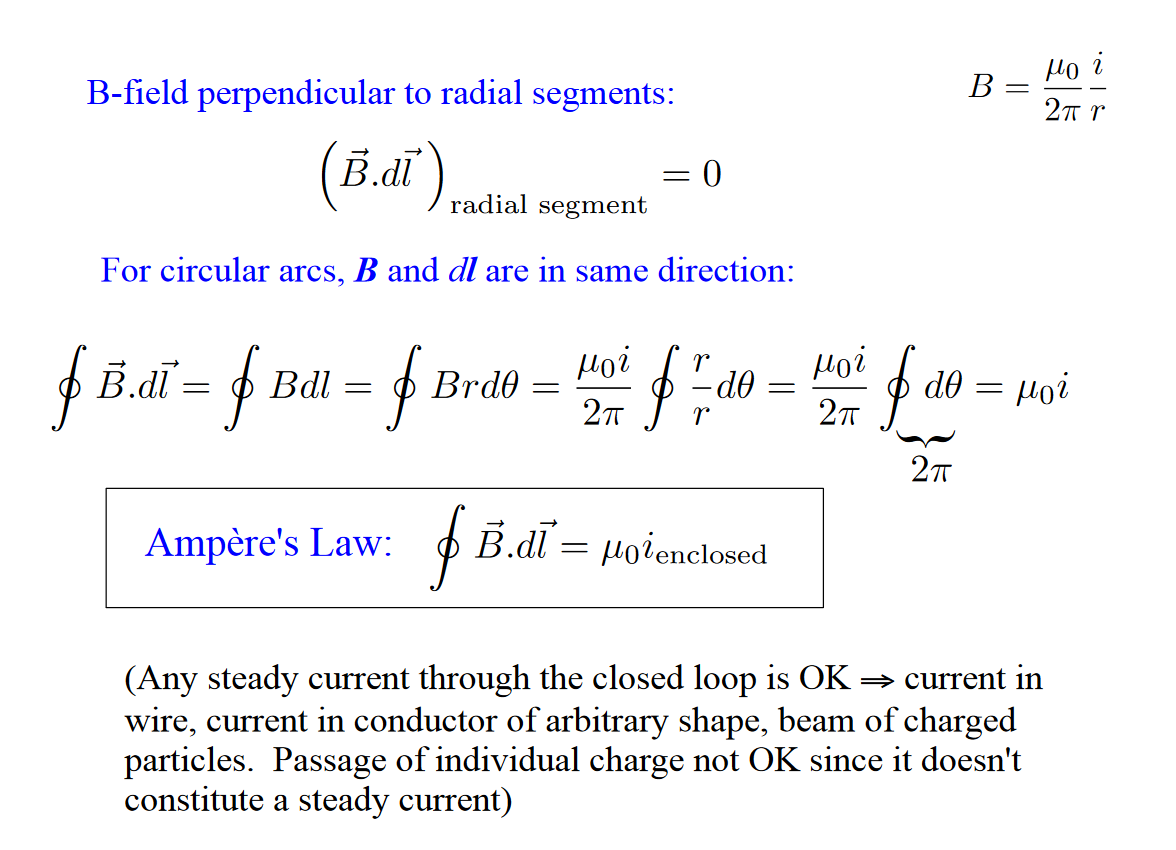
Just like how Gauss’ Law makes it easy to solve complex charge distributions, Ampère’s Law simplifies calculating magnetic fields for current flowing through complex shapes.
#todo Applications!!
Definition
Assume we define a closed loop around some current carrying object. Then:
Formula
Ampère's Law
\oint \vec{B} \cdot d\vec{l} = \mu_{0} I_{enclosed}
##### Terms
* $\vec{B}$ = [Magnetic Field](Magnetic%20Field.md) (in $T$)
* $\vec{l}$ = Path vector of the loop. $d\vec{l}$ returns an infinitesimally small section of said path.
* $\mu_{0}$ = Permeability of free space
* $I_{enclosed}$ = [Current](Current.md) enclosed inside the loop.
* $\oint$ = Line integral
>[!info] Unfinished...
>
>This law is very useful in obtaining the relationship between current and magnetic fields, but there is another way a magnetic field can be created - through a changing [Electric Field](electric%20field.md)! The addition is shown in the revised [Ampère-Maxwell Law](Ampère-Maxwell%20Law.md).
### Derivation
Ampere's Law is derived from the [Biot-Savart Law](Biot-Savart%20Law.md), when applied through a [Infinite Line of Current](Infinite%20Line%20of%20Current.md).
The formula is given by:
Observe what happens if we decide to take a circular loop around the wire:
The circumference of this loop is given by: $C = 2\pi r$. And if we define this loop to be made up of infinite $d\vec{l}$ then:
\oint d\vec{l} = C = 2\pi r
\oint \vec{B} \cdot d\vec{l} = \dfrac{\mu_{0}}{2\pi} \dfrac{I}{r} \times C = \mu_{0}I
Now let's look at a more complex loop:
This loop consists of areas where $\vec{B} \perp \vec{l}$ (in red) and $\vec{B} \parallel \vec{l}$ (in green):
#TODO 